Playing with coupled oscillators - v3
Contents
Playing with coupled oscillators - v3#
This notebook builds on previous notebooks such as Orbit_games.ipynb to implement the solution of linear coupled differential equations using solve_ivp as well as with more analytic methods. The formalism and notation is based on Taylor, Chapter 11: Coupled Oscillators and Normal Modes.
The general form of the equations to solve is compactly expressed in matrix form:
\(\begin{align} \mathbf{M} \mathbf{\ddot q} = - \mathbf{K} \mathbf{q} \end{align}\)
where \(\mathbf{M}\) and \(\mathbf{K}\) are \(N \times N\) symmetric matrices and \(\mathbf{q}\) is a vector of generalized coordinates (this is \(\mathbf{x}\) in the beginning discussion in Taylor).
We can solve this directly as differential equations or solve for normal modes. For the former, we map the problem onto linear differential equations and the vectors \(\mathbf{y}\) and \(d\mathbf{y}/dt\), which are each of length \(2N\). Here is the \(N=2\) mapping:
\(\begin{align} \mathbf{y} = \left( \begin{array}{c} q_1 \\ q_2 \\ \dot q_1 \\ \dot q_2 \end{array} \right) \qquad\qquad \frac{d\mathbf{y}}{dt} = \left( \begin{array}{c} \dot q_1 \\ \dot q_2 \\ \ddot q_1 \\ \ddot q_2 \end{array} \right) \end{align}\)
Then for the \(\ddot q\) parts we use the matrix solution of our equation:
\(\begin{align} \mathbf{\ddot q} = - \mathbf{M}^{-1} \mathbf{K} \mathbf{q} \end{align}\)
where \(\mathbf{M}^{-1}\) means the matrix inverse. Check out the implementation below!
To solve for normal modes, we solve the generalized eigenvalue problem
\(\begin{align} (\mathbf{K} - \omega^2 \mathbf{M}) \mathbf{a} = 0 \end{align}\)
using functions from scipy.linalg to find the eigenvalues and eigenvectors. We use these below together with the numerical solutions to make plots of the normal modes.
import numpy as np
import matplotlib.pyplot as plt
from scipy.integrate import solve_ivp
from scipy import linalg as LA
# Change the common font size
font_size = 14
plt.rcParams.update({'font.size': font_size})
class CoupledOscillators:
"""
Solve the equations for linear coupled oscillators in matrix formulation.
"""
def __init__(self, M_matrix, K_matrix, b_matrix=np.zeros(1)):
self.M_matrix = M_matrix
self.K_matrix = K_matrix
self.q_len = len(self.M_matrix)
if b_matrix.any():
self.b_matrix = b_matrix
else:
self.b_matrix = np.zeros((self.q_len))
self.full_matrix = np.zeros((self.q_len, self.q_len))
def dy_dt(self, t, y):
"""
This function returns the right-hand side of the diffeq:
[dq_vec/dt d^2q_vec/dt^2]
Parameters
----------
t : float
time
y : float
vector with y[:q_len] = q_vec, y[q_len:] = dqvec/dt
"""
dy_dt_vec = np.zeros(2*self.q_len) # Initialize to twice q_vec size
# Set the upper half [:self.q_len] of dy_dt to the lower half of y
dy_dt_vec[:self.q_len] = y[self.q_len:]
# Set the lower half [self.q_len:] of dy_dt to the vector that
# results from M^{-1} K y where @ is matrix multiplication
dy_dt_vec[self.q_len:] = -1.* LA.inv(self.M_matrix) @ \
( self.K_matrix @ y[:self.q_len] + \
self.b_matrix @ y[self.q_len:] )
return dy_dt_vec
def solve_ode(self, t_pts, q_vec_0, q_vec_dot_0,
method='RK45',
abserr=1.0e-10, relerr=1.0e-10):
"""
Solve the ODE given initial conditions.
Use solve_ivp with the option of specifying the method.
Specify smaller abserr and relerr to get more precision.
"""
y = np.concatenate((q_vec_0, q_vec_dot_0))
solution = solve_ivp(self.dy_dt, (t_pts[0], t_pts[-1]),
y, t_eval=t_pts, method=method,
atol=abserr, rtol=relerr)
q_vec, q_vec_dot = np.array_split(solution.y, 2)
return q_vec, q_vec_dot
def find_eigenmodes(self):
"""
Find the normal modes.
"""
eig_vals, eig_vecs = LA.eigh(K_matrix, M_matrix)
self.frequencies = np.sqrt(eig_vals)
return eig_vals, eig_vecs
def plot_eigenmodes(self, t_pts, output_file=None):
"""
plot the normal modes separately
"""
from itertools import cycle
colors = ['blue', 'green', 'red', 'purple']
eig_vals, eig_vecs = self.find_eigenmodes()
for i in np.arange(self.q_len):
q_vec_0 = eig_vecs[:,i] # this is the i'th eigenvector
q_vec_dot_0 = np.zeros(self.q_len) # start with all qdots = 0
q_vec, q_vec_dot = self.solve_ode(t_pts, q_vec_0, q_vec_dot_0)
fig, axes = plt.subplots(self.q_len, 1,
figsize=(10, 2.*self.q_len))
color_list = cycle(colors)
for j in np.arange(self.q_len):
axes[j].plot(t_pts, q_vec[j], color=next(color_list))
axes[j].set_xlabel(r'$t$')
axes[j].set_ylabel(fr'$q_{j+1:d}$')
axes[j].axvline(2.*np.pi/np.sqrt(eig_vals[i]),
color='black', linestyle=':')
overall_title = fr'Normal mode {i+1:d}, ' + \
fr'$\omega = {self.frequencies[i]:.2f}$'
fig.suptitle(overall_title, va='center')
fig.tight_layout()
if output_file:
output_file_name = output_file.replace('.', f'_{i:d}.', 1)
fig.savefig(output_file_name, dpi=200, bbox_inches='tight')
def start_stop_indices(t_pts, plot_start, plot_stop):
start_index = (np.fabs(t_pts-plot_start)).argmin() # index in t_pts array
stop_index = (np.fabs(t_pts-plot_stop)).argmin() # index in t_pts array
return start_index, stop_index
Section 11.2#
m = 1.
M_matrix = np.array([ [m, 0.], [0., m] ])
print('M matrix: \n', M_matrix, '\n')
k = 1.
K_matrix = np.array([ [2.*k, -k], [-k, 2.*k]])
print('K matrix: \n', K_matrix, '\n')
# b = 0
# b_matrix = np.array([ [b, 0], [0, b]])
# print('b matrix: \n', b_matrix, '\n')
co1 = CoupledOscillators(M_matrix, K_matrix)
eig_vals, eig_vecs = co1.find_eigenmodes() # LA.eigh(K_matrix, M_matrix)
print(f'omega^2 = {eig_vals}')
print(f'frequencies = {co1.frequencies}')
M matrix:
[[1. 0.]
[0. 1.]]
K matrix:
[[ 2. -1.]
[-1. 2.]]
omega^2 = [1. 3.]
frequencies = [1. 1.73205081]
print(eig_vecs[:,0])
print(eig_vecs[:,1])
[-0.70710678 -0.70710678]
[-0.70710678 0.70710678]
t_start = 0.
t_end = 10.
delta_t = 0.001
t_pts = np.arange(t_start, t_end+delta_t, delta_t)
co1.plot_eigenmodes(t_pts, 'Section11p2.png')
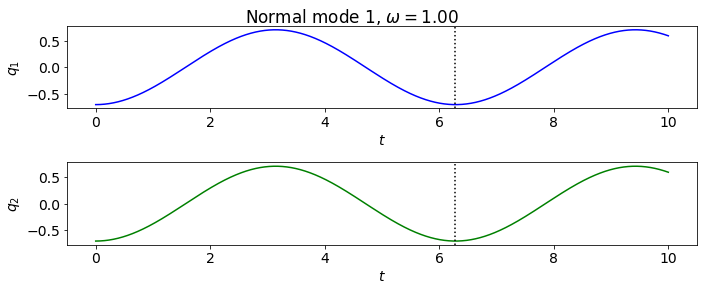
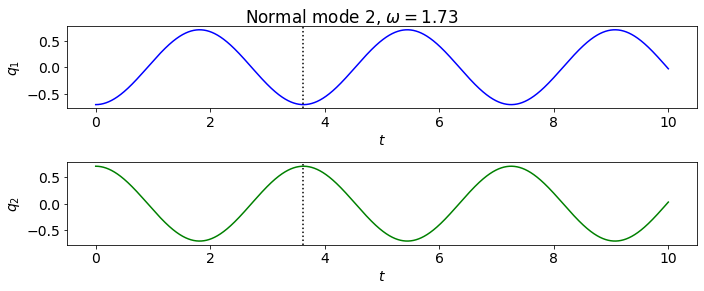
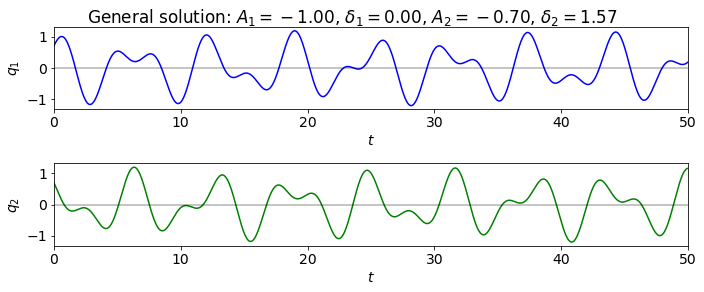
Figure 11.6#
This has \(A_1 = 1\), \(A_2 = 0.7\), \(\delta_1 = 0\) and \(\delta_2 = \pi/2\).
t_start = 0.
t_end = 50.
delta_t = 0.001
t_pts = np.arange(t_start, t_end+delta_t, delta_t)
colors = ['blue', 'green', 'red', 'purple']
# changing A_i -> -A_i to match convention in Figure 11.6
A_1 = -1.
delta_1 = 0.
A_2 = -0.7
delta_2 = np.pi/2.
omega_1 = co1.frequencies[0]
omega_2 = co1.frequencies[1]
q_vec_0 = A_1 * eig_vecs[:,0] * np.cos(-delta_1) + \
A_2 * eig_vecs[:,1] * np.cos(-delta_2)
q_vec_dot_0 = omega_1 * A_1 * eig_vecs[:,0] * np.sin(delta_1) + \
omega_2 * A_2 * eig_vecs[:,1] * np.sin(delta_2)
q_vec_11p6, q_vec_dot_11p6 = co1.solve_ode(t_pts, q_vec_0, q_vec_dot_0)
fig, axes = plt.subplots(co1.q_len, 1, figsize=(10, 2.*co1.q_len))
for j in np.arange(co1.q_len):
axes[j].plot(t_pts, q_vec_11p6[j], color=colors[j])
axes[j].set_xlabel(r'$t$')
axes[j].set_ylabel(fr'$q_{j+1:d}$')
axes[j].set_xlim(t_pts[0], t_pts[-1])
axes[j].axhline(0., color='black', alpha=0.3)
overall_title = 'General solution: ' + fr'$A_1 = {A_1:.2f}$, ' + \
fr'$\delta_1 = {delta_1:.2f}$, ' + \
fr'$A_2 = {A_2:.2f}$, ' + \
fr'$\delta_2 = {delta_2:.2f}$'
fig.suptitle(overall_title, va='center')
fig.tight_layout()
print( q_vec_0, q_vec_dot_0 ) # debugging check
[0.70710678 0.70710678] [ 0.85732141 -0.85732141]
Problem 11.5#
Almost the same as section 11.2, but mass m2 is not attached by a spring to the wall.
m = 1.
M_matrix = np.array([ [m, 0.], [0., m] ])
print('M matrix: \n', M_matrix, '\n')
k = 1.
K_matrix = np.array([ [2.*k, -k], [-k, k]])
print('K matrix: \n', K_matrix, '\n')
# b = 0
# b_matrix = np.array([ [b, 0], [0, b]])
# print('b matrix: \n', b_matrix, '\n')
co1 = CoupledOscillators(M_matrix, K_matrix)
eig_vals, eig_vecs = co1.find_eigenmodes() # LA.eigh(K_matrix, M_matrix)
print(f'omega^2 = {eig_vals}')
print(f'frequencies = {co1.frequencies}')
M matrix:
[[1. 0.]
[0. 1.]]
K matrix:
[[ 2. -1.]
[-1. 1.]]
omega^2 = [0.38196601 2.61803399]
frequencies = [0.61803399 1.61803399]
print(eig_vecs[:,0])
print(eig_vecs[:,1])
[-0.52573111 -0.85065081]
[-0.85065081 0.52573111]
t_start = 0.
t_end = 10.
delta_t = 0.001
t_pts = np.arange(t_start, t_end+delta_t, delta_t)
co1.plot_eigenmodes(t_pts)

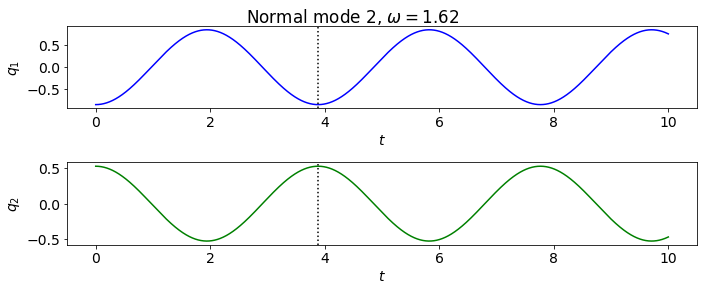
Problem 11.7#
Writing the general solution to the cart problem in Section 11.2 in a different form and plot two solutions.
m = 1.
M_matrix = np.array([ [m, 0.], [0., m] ])
print('M matrix: \n', M_matrix, '\n')
k = 1.
K_matrix = np.array([ [2.*k, -k], [-k, 2.*k]])
print('K matrix: \n', K_matrix, '\n')
# b = 0
# b_matrix = np.array([ [b, 0], [0, b]])
# print('b matrix: \n', b_matrix, '\n')
co1 = CoupledOscillators(M_matrix, K_matrix)
eig_vals, eig_vecs = co1.find_eigenmodes() # LA.eigh(K_matrix, M_matrix)
print(f'omega^2 = {eig_vals}')
print(f'frequencies = {co1.frequencies}')
M matrix:
[[1. 0.]
[0. 1.]]
K matrix:
[[ 2. -1.]
[-1. 2.]]
omega^2 = [1. 3.]
frequencies = [1. 1.73205081]
t_start = 0.
t_end = 30.
delta_t = 0.001
t_pts = np.arange(t_start, t_end+delta_t, delta_t)
colors = ['blue', 'green', 'red', 'purple']
# changing A -> -A to match convention in Taylor
A = -1.
B_1 = A
B_2 = 0.
C_1 = 0.
C_2 = 0.
omega_1 = co1.frequencies[0]
omega_2 = co1.frequencies[1]
q_vec_0 = B_1 * eig_vecs[:,0] + \
B_2 * eig_vecs[:,1]
q_vec_dot_0 = omega_1 * C_1 * eig_vecs[:,0] + \
omega_2 * C_2 * eig_vecs[:,1]
q_vec_11p7a, q_vec_dot_11p7a = co1.solve_ode(t_pts, q_vec_0, q_vec_dot_0)
fig, axes = plt.subplots(co1.q_len, 1, figsize=(10, 2.*co1.q_len))
for j in np.arange(co1.q_len):
axes[j].plot(t_pts, q_vec_11p7a[j], color=colors[j])
axes[j].set_xlabel(r'$t$')
axes[j].set_ylabel(fr'$q_{j+1:d}$')
axes[j].set_xlim(t_pts[0], t_pts[-1])
axes[j].axhline(0., color='black', alpha=0.3)
overall_title = 'Specific solution 1: ' + fr'$B_1 = {B_1:.2f}$, ' + \
fr'$C_1 = {C_1:.2f}$, ' + \
fr'$B_2 = {B_2:.2f}$, ' + \
fr'$C_2 = {C_2:.2f}$'
fig.suptitle(overall_title, va='center')
fig.tight_layout()
print( q_vec_0, q_vec_dot_0 ) # debugging check
[0.70710678 0.70710678] [-0. 0.]
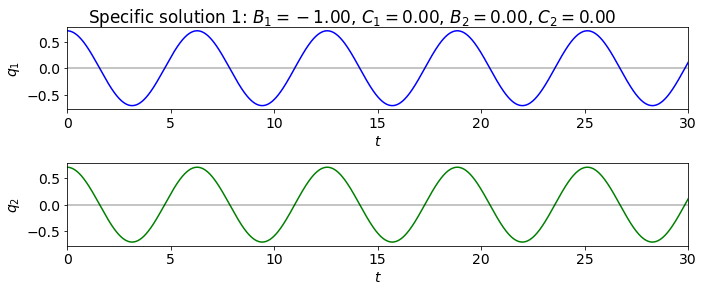
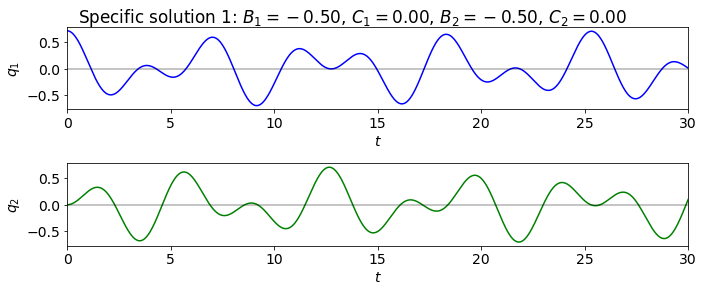
colors = ['blue', 'green', 'red', 'purple']
# changing A -> -A to match convention in Taylor
A = -1.
B_1 = A / 2.
B_2 = A / 2.
C_1 = 0.
C_2 = 0.
omega_1 = co1.frequencies[0]
omega_2 = co1.frequencies[1]
q_vec_0 = B_1 * eig_vecs[:,0] + \
B_2 * eig_vecs[:,1]
q_vec_dot_0 = omega_1 * C_1 * eig_vecs[:,0] + \
omega_2 * C_2 * eig_vecs[:,1]
q_vec_11p7a, q_vec_dot_11p7a = co1.solve_ode(t_pts, q_vec_0, q_vec_dot_0)
fig, axes = plt.subplots(co1.q_len, 1, figsize=(10, 2.*co1.q_len))
for j in np.arange(co1.q_len):
axes[j].plot(t_pts, q_vec_11p7a[j], color=colors[j])
axes[j].set_xlabel(r'$t$')
axes[j].set_ylabel(fr'$q_{j+1:d}$')
axes[j].set_xlim(t_pts[0], t_pts[-1])
axes[j].axhline(0., color='black', alpha=0.3)
overall_title = 'Specific solution 1: ' + fr'$B_1 = {B_1:.2f}$, ' + \
fr'$C_1 = {C_1:.2f}$, ' + \
fr'$B_2 = {B_2:.2f}$, ' + \
fr'$C_2 = {C_2:.2f}$'
fig.suptitle(overall_title, va='center')
fig.tight_layout()
print( q_vec_0, q_vec_dot_0 ) # debugging check
[0.70710678 0. ] [-0. 0.]
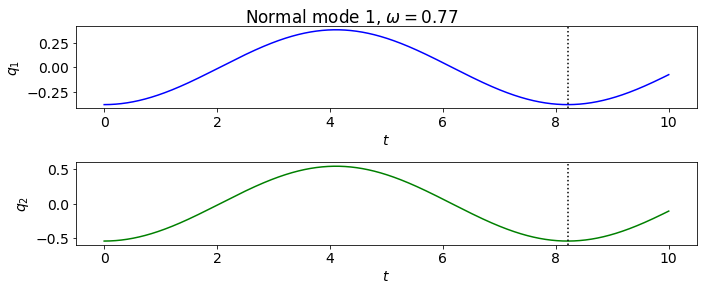
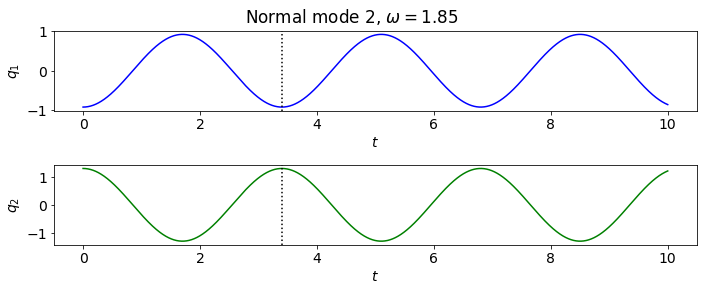
Double pendulum with small oscillations#
m = 1.
L = 1.
M_matrix = m * L**2 * np.array([ [2., 1.], [1., 1.] ])
print('M matrix: \n', M_matrix, '\n')
k = 1.
omega_0 = 1.
K_matrix = m * L**2 * np.array([ [2.*omega_0**2, 0.], [0., omega_0**2]])
print('K matrix: \n', K_matrix, '\n')
# b = 0
# b_matrix = np.array([ [b, 0], [0, b]])
# print('b matrix: \n', b_matrix, '\n')
double_pendulum = CoupledOscillators(M_matrix, K_matrix)
eig_vals, eig_vecs = double_pendulum.find_eigenmodes()
print(f'omega^2 = {eig_vals}')
print(f'frequencies = {double_pendulum.frequencies}')
print(f'{2. + np.sqrt(2.):.4f} {2. - np.sqrt(2.):.4f}')
M matrix:
[[2. 1.]
[1. 1.]]
K matrix:
[[2. 0.]
[0. 1.]]
omega^2 = [0.58578644 3.41421356]
frequencies = [0.76536686 1.84775907]
3.4142 0.5858
print(eig_vecs[:,0])
print(eig_vecs[:,1])
[-0.38268343 -0.5411961 ]
[-0.92387953 1.30656296]
t_start = 0.
t_end = 10.
delta_t = 0.001
t_pts = np.arange(t_start, t_end+delta_t, delta_t)
double_pendulum.plot_eigenmodes(t_pts)